Hei! 45+ Lister over Unit 8 Right Triangles And Trigonometry Key? Ccss.math.content.5.nf.b.4.b find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths.

Unit 8 Right Triangles And Trigonometry Key | Chapter 8 further applications of trigonometry. In this section, we will extend those definitions so that we can apply them to right triangles. In earlier sections, we used a unit circle to define the trigonometric functions. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. This program calculates answers for right triangles, given two pieces of information.

In this section, we will extend those definitions so that we can apply them to right triangles. Chapter 8 further applications of trigonometry. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. In earlier sections, we used a unit circle to define the trigonometric functions. This program calculates answers for right triangles, given two pieces of information.

Gina Wilson Unit 8 Right Triangles And Trigonometry Pdf Free Download from vibdoc.com. Finn ut mer her.

Using right triangles to evaluate trigonometric functions. Recognize right triangles as a category, and identify right triangles. In this section, we will extend those definitions so that we can apply them to right triangles. Chapter 8 further applications of trigonometry. In earlier sections, we used a unit circle to define the trigonometric functions. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. Introduction to further applications of trigonometry; Ccss.math.content.5.nf.b.4.b find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths.

Ccss.math.content.5.nf.b.4.b find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Using right triangles to evaluate trigonometric functions. The value of the sine or cosine function of latext/latex is its value at latext/latex radians. Recognize right triangles as a category, and identify right triangles. In this section, we will extend those definitions so that we can apply them to right triangles. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. Introduction to further applications of trigonometry; It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. This program calculates answers for right triangles, given two pieces of information. In earlier sections, we used a unit circle to define the trigonometric functions. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. Chapter 8 further applications of trigonometry. 8.5 polar form of complex.

Using right triangles to evaluate trigonometric functions. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. Introduction to further applications of trigonometry; Recognize right triangles as a category, and identify right triangles. Oct 23, 2014 · key terms;

Solving For Missing Angles Algebra 1 from img.yumpu.com. Finn ut mer her.

Introduction to further applications of trigonometry; Chapter 8 further applications of trigonometry. Recognize right triangles as a category, and identify right triangles. In this section, we will extend those definitions so that we can apply them to right triangles. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. Using right triangles to evaluate trigonometric functions. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. In earlier sections, we used a unit circle to define the trigonometric functions.

Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. Recognize right triangles as a category, and identify right triangles. Using right triangles to evaluate trigonometric functions. Chapter 8 further applications of trigonometry. Oct 23, 2014 · key terms; Ccss.math.content.5.nf.b.4.b find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Introduction to further applications of trigonometry; Multiply fractional side lengths to find areas of rectangles, and represent fraction products. In earlier sections, we used a unit circle to define the trigonometric functions. 8.5 polar form of complex. This program calculates answers for right triangles, given two pieces of information. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. The value of the sine or cosine function of latext/latex is its value at latext/latex radians.

Oct 23, 2014 · key terms; This program calculates answers for right triangles, given two pieces of information. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. The value of the sine or cosine function of latext/latex is its value at latext/latex radians. 8.5 polar form of complex.

Right Triangle Trigonometry Guided Notes For Right Triangles Unit Geometry from ecdn.teacherspayteachers.com. Finn ut mer her.

This program calculates answers for right triangles, given two pieces of information. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. Introduction to further applications of trigonometry; Recognize right triangles as a category, and identify right triangles. The value of the sine or cosine function of latext/latex is its value at latext/latex radians. Oct 23, 2014 · key terms; Using right triangles to evaluate trigonometric functions. In earlier sections, we used a unit circle to define the trigonometric functions.

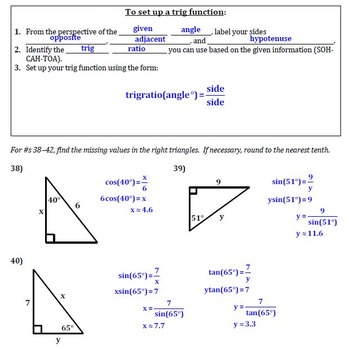
In this section, we will extend those definitions so that we can apply them to right triangles. Using right triangles to evaluate trigonometric functions. The value of the sine or cosine function of latext/latex is its value at latext/latex radians. In earlier sections, we used a unit circle to define the trigonometric functions. Introduction to further applications of trigonometry; This program calculates answers for right triangles, given two pieces of information. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. 8.5 polar form of complex. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. Oct 23, 2014 · key terms; Recognize right triangles as a category, and identify right triangles. Chapter 8 further applications of trigonometry. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$.

Unit 8 Right Triangles And Trigonometry Key: Ccss.math.content.5.nf.b.4.b find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths.

Source: i.pinimg.com | Se mer informasjon.

The value of the sine or cosine function of latext/latex is its value at latext/latex radians. Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. Using right triangles to evaluate trigonometric functions. 8.5 polar form of complex. Recognize right triangles as a category, and identify right triangles.

Source: us-static.z-dn.net| Se mer informasjon.

Recognize right triangles as a category, and identify right triangles. It uses the getkey function to store user input into a string, one number at a time, and displays it on the graph screen as the user enters it, one number at a time until the enter key is pressed. Using right triangles to evaluate trigonometric functions.

Source: media.cheggcdn.com| Se mer informasjon.

Problem 7 identify the hypotenuse , and the opposite and adjacent sides of $$ \angle bac $$. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. Oct 23, 2014 · key terms;

Source: i.ytimg.com| Se mer informasjon.

Using right triangles to evaluate trigonometric functions. Multiply fractional side lengths to find areas of rectangles, and represent fraction products. Oct 23, 2014 · key terms;